
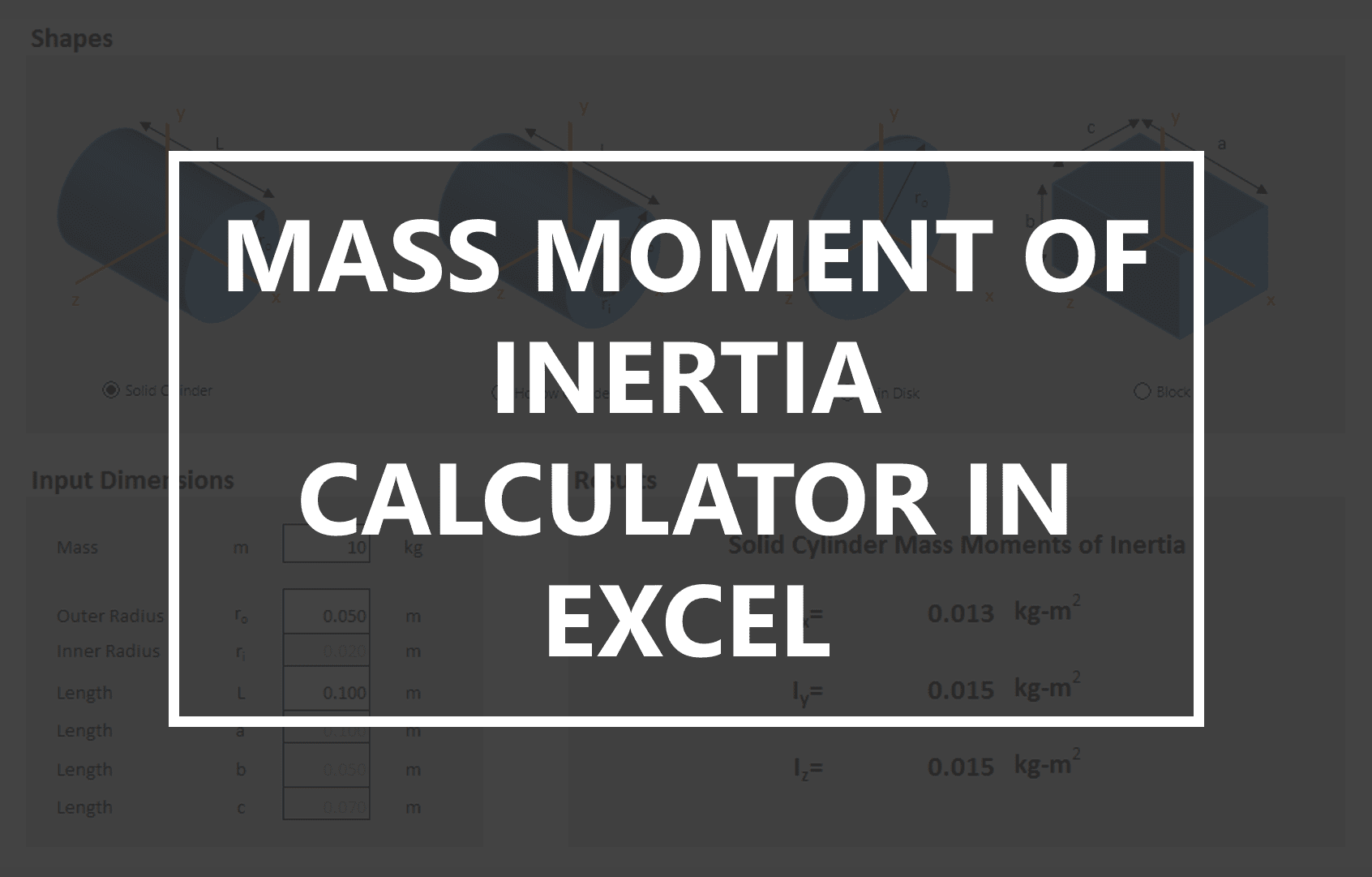
The moment of inertia of an object refers to a calculated measure for any given rigid body that’s rotating around a fixed axis.
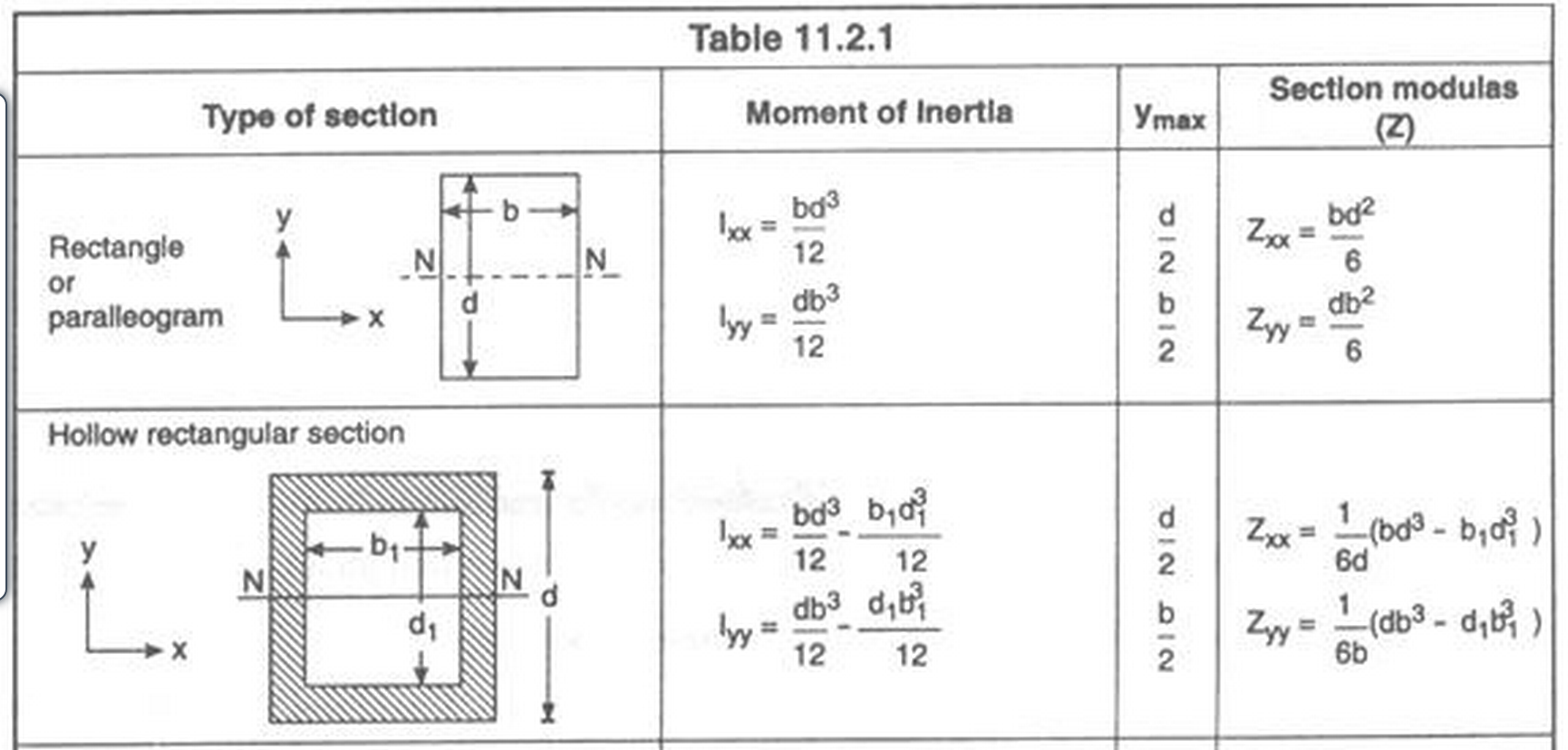
Elastic Section Moduli: The elastic section moduli are equal to the second moments of area / moments of inertia divided by the distance to the farthest fibre in the cross-section perpendicular to the axis of bending. Integrating curvatures over beam length, the deflection, at some point along x-axis, should also be reversely proportional to I. You can find the moment of inertia of an object using this area moment of inertia calculator. The Product Moment of Inertia is, by definition, zero for principal axes. Therefore, it can be seen from the former equation, that when a certain bending moment M is applied to a beam cross-section, the developed curvature is reversely proportional to the moment of inertia I. Where Ixy is the product of inertia, relative to centroidal axes x,y, and Ixy' is the product of inertia, relative to axes that are parallel to centroidal x,y ones, having offsets from them d_. Please use consistent units for any input. The calculated results will have the same units as your input. Enter the shape dimensions h, b, tfand twbelow. Solve for the moment of inertia using the transfer formula. This tool calculates the moment of inertia I (second moment of area) of an I/H section (also called W-beam or double-T). This calculator will calculate the Moment of Inertia of a bar rotating around its centre and rotating around its end, a cylinder or disc rotating around its.
#MOMENT OF INERTIA CALCULATOR FREE#
Location of centroid of the compound shape from the axes x 76000 / 2800 x 27.143 mm y 84000 / 2800 y 30 mm b. Moment of Inertia Calculator is a free online tool that displays the moment of inertia for the given mass and distance. Solve for the centroid of the whole complex shape by tabulating the solution. Where I' is the moment of inertia in respect to an arbitrary axis, I the moment of inertia in respect to a centroidal axis, parallel to the first one, d the distance between the two parallel axes and A the area of the shape (=bh in case of a rectangle).įor the product of inertia Ixy, the parallel axes theorem takes a similar form: Solving for the Moment of Inertia of Compound Shapes John Ray Cuevas Solution a. The so-called Parallel Axes Theorem is given by the following equation:
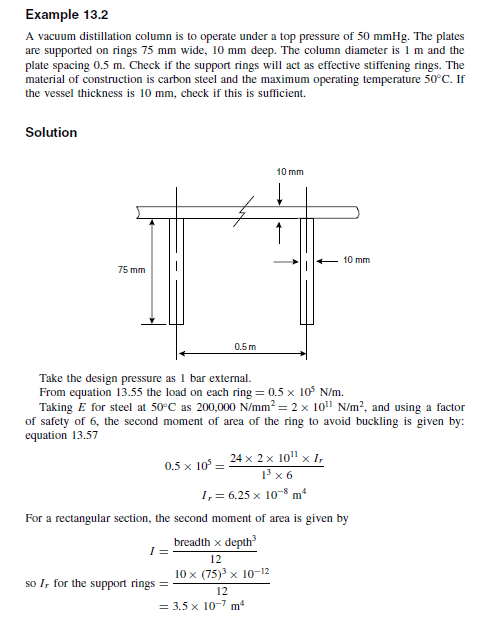
The moment of inertia of any shape, in respect to an arbitrary, non centroidal axis, can be found if its moment of inertia in respect to a centroidal axis, parallel to the first one, is known.


 0 kommentar(er)
0 kommentar(er)
